by John P. Pratt (21 Dec 2012)
©2012 by John P. Pratt. All rights Reserved.
| 1. Musical Scales |
| 1.1 The Octave |
| 1.2 Pythagorean Scale |
| 1.3 Diatonic Scale |
| 1.4 Seventh Chord |
| 1.5 Chromatic Scale |
| 1.6 440 or 432 Hz? |
| 2. The Wholesome Scale |
| 3. The Guit-harp |
| 4. Conclusion |
| Notes |
Why are there seven notes in the usual musical scale: do, re, mi, fa, so, la, and ti? Why not nine or ten? Why are some of the keys on a piano white and some are black? Are there different kinds of scales? Do some scales sound better than others? This paper proposes that one scale is more natural and melodic because it is based on how actual strings vibrate. However, it is rarely used. Then it is shown that it can be defined to have integer (whole number) values for all frequencies over the range of the keys on a piano. Two of those values have been proposed as the best frequencies on which to base a musical scale. So let's look at the basics of how string music is made, and what notes one hears naturally.
Let's look at three principal musical scales, all of which contain the notes do, re, mi, fa, so, la, and ti. That means there are three different ways to define exactly what is meant by how each note sounds relative to the others and what is the spacing between them. Let's look at each of them in order to compare their merits.
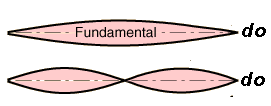
Let's begin by considering the octave. The name octave comes from the fact that on the scale of seven notes, the eighth note sounds so similar to the first, that it is given the same name, and said to be an "octave" higher ("oct" meaning eight). Thus we often think of the scale as eight notes rather than seven: do, re, mi, fa, so, la, ti, do. Note that the first and last notes are both do. That is because in some very important way, they sound the same.
 |
Now consider what happens if the string is plucked about a quarter of the way along, rather than in the center. It will vibrate with at least two frequencies as shown in the lower picture in Figure 1. In addition to the fundamental pattern, the string will also vibrate as if the center of it were holding still. That makes it vibrate at twice the frequency. But no matter where it is plucked, the string will always vibrate mostly at the fundamental frequency. So the effect is that one will hear both the fundamental do note, and also another note of twice the frequency. Because they are usual heard together, and sound so similar, they are given the same name do. The one at twice the frequency is said to be an "octave" higher. It is also called the "first harmonic" of the fundamental frequency.
 |
All three of the musical scales reviewed in this article are based on the octave being exactly a factor of two in frequency. So now we have our first note: do. Now let's look at three different ways to determine the other seven notes.
Now let's look at how to find other natural notes that are also heard when a string is plucked. Look again at the guitar in Figure 2. Notice that the strings are not plucked (or strummed) near the middle of the strings, but closer to two thirds of the way down. What effect will that have?
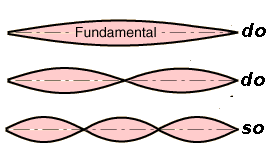 |
Think about that. Whenever a string is plucked, one hears not only the fundamental note that we are calling do, but also another do an octave higher, and then the note so in that octave. To me this is a huge point in choosing what the notes in a scale should be. The notes that are harmonics are serious contenders to be defined to be the notes of a scale, rather that just some arbitrary frequency.
A common way to talk about frequencies is to list them as ratios of their frequency to the frequency of the base note do. Our ears hear ratios of frequencies as equivalent changes in pitch. The octave is a good example. A note that is three octaves higher than another has 8 times the frequency (2 x 2 x 2), not six times (2 + 2 + 2).
In this case, the note so will always have a frequency of 3/2 or 1.5 times the note do of that octave. Looking at Figure 3, the frequency of the second harmonic so to the first harmonic (its do) is 3/2 or exactly 1.5.
Long ago someone got the idea of putting two strings on an instrument. The first can be tuned to any do note of choice, and the second to its so note. Now whenever the first string is plucked, not only will a so note be heard as a harmonic, but it will also cause the so string to vibrate slightly. If the two are plucked together, the sound is very pleasing. That locks in so as a desired note on a scale.
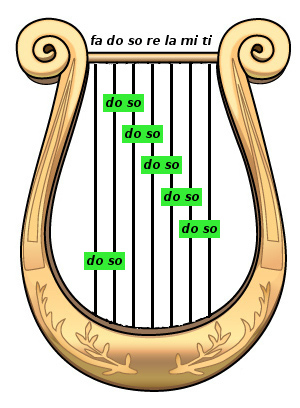 |
One can repeat this process to see if it works, and leads to pleasing notes. The answer is that it can be repeated at least three more times to get six notes of a scale (See Figure 4). As it is repeated, it is noticed that these notes are somewhat equally spaced around all the previous notes in all the octaves. For example, consider the note re that we just found. The ratio of re to do is 9/8. Nearly that exact ratio just keeps showing up over and over as we go around different octaves. It is what is often called one "whole step" between notes. As explained before, a whole step is really a ratio of two frequencies. So the bottom line here is that re is the note that follows do, being one whole step above it.
The first six notes found in this manner are do, so, re, la, mi, and ti. With this last note, it is noticed that it is only about a half step below the do just above it, meaning about half the ratio of a whole step. So should ti be accepted as a note? The answer is all in the sound and the answer is definitely yes, so the note ti is accepted as valid. But now the scale includes both whole and half steps.
|
The other choice is to perform the note finding procedure in the opposite direction. That is, look at the original note and consider it to be the so of a lower string. In that case, fa turns out to be a half step above mi and a whole step below so. That turns out to be preferable because of how it sounds and how chords are made, which is covered in the next section. That completes the process of finding the seven notes. In Figure 4, the notes are written above the seven strings.
This scale is called the Pythagorean scale, supposedly because it was either discovered or preferred by that great ancient Greek scholar. The scale has all whole step intervals (really ratios) except for between mi and fa, and also between ti and do. One really nice mathematical point is that while the whole steps vary slightly from each other, both the half steps are exactly the same ratio.
Just for the record the frequency ratios for the notes on the Pythagorean scale are listed in Table 1. It is left as an exercise for the reader to derive all those values by the method discussed, and also to show that the two half steps are identical.
 |
We already defined a mi note in the Pythagorean scale. It was found by successive applying of the do-so relationship. How different are these two notes? This new mi has a base frequency (in first octave) of 5/4, or 1.250. The Pythagorean mi has a frequency of 81/64, or 1.266. Which is better?
Before even determining the rest of the scale, here is one vote for the new mi. It is actually heard as a natural harmonic when a string is played. The Pythagorean mi would be heard when the fourth string in a succession might be played (See Fig. 4), which would be rare. That alone doesn't prove the new one sounds better, but only that it is a natural note that is heard whenever a string is plucked. Before deciding based on theory alone, let's look at how the other notes are derived.
 |
|
So again we can ask which of the two values for those three notes are better?
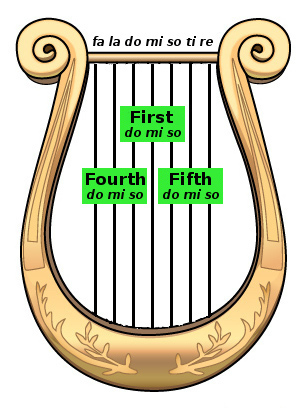
Many simple songs are based on a progression of three cords, all of them being "triads" A triad (technically a "major triad") is the do-mi-so combination of notes. The first chord is a triad starting with do, the second chord starts on the fourth note fa, and the final triad begins on the fifth note so. In this article those chords are called the First, Fourth, and Fifth chords. They are also labeled in some notations with Roman numerals I, IV, V. The fancy names are "tonic", "subdominant" and "dominant" chords. The important thing is that they form the basis of a lot of music.
Now look again at Figure 6. Notice that the three triads that were used to determine the diatonic scale just happen to be the exact same three triads that so much music is based on. To me that is a compelling reason to choose those triads to be the basis of a musical scale.
One more point is that in the Pythagorean scale, a decision had to be made when picking the notes fa and ti. In this scheme, there is no question because the triads are used so much in real music. One more nice feature can be verified from the frequency values in Table 2. Namely, again it turns out that the two half-steps in the scale have exactly the same value as each other. That seems surprising because we changed the values for mi and ti, but in such a way that the interval between them and the next higher note (ratio of 16/15) is the same for both of these new notes.
Before looking at the chromatic scale, let's consider a finer detail of music. If the reader is already bored to tears, the next section could be skipped without missing much.
Anyone who plays the guitar knows that in real life, even though the simple music theory suggests that the three basic chords ought to be the First, Fourth, and Fifth chords, there is something that just sounds a lot better. It is called a "Seventh", and it just sounds "right" and creates a feeling of transition back to the First chord.
 |
The three basic chords for simple songs should then be the major triads of the First note (C), the Fourth Note (F), and the Fifth note (G), and then returning to C. So the progression would be the chords C, F, G, C. In other words, the entire song can be sung by just using those three chords. At least in simple theory.
In practice, that is actually a rare case in most folk songs, at least those back in the fifties and sixties. So what three chords are they based on? Most folk songs sound much better by playing the chord G7 instead of G in that series. That is, many songs can be played with just the three chords C, F and G7.
So what is a seventh? It is formed by adding one more note to the major triad. That note is about halfway between la and ti. That means it is not even one of the notes on our diatonic scale! Or is it? Let's look at the example in the key of C.
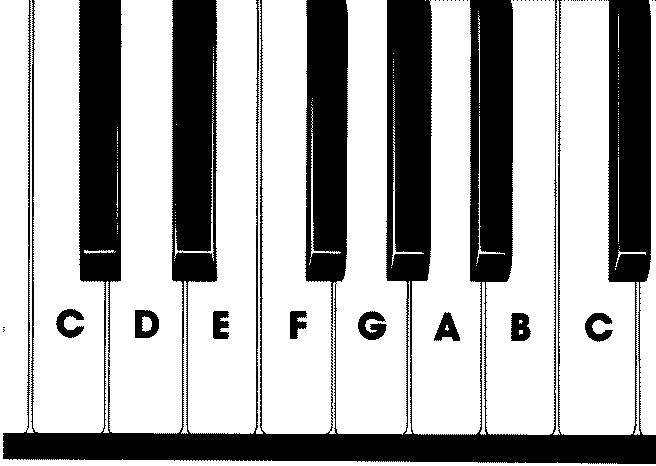
In the G chord, the triad is formed by the notes G, B, and D. Now in the key of G (meaning G is do), the note ti is only a half step below G, so it is not F, which is a whole note lower. On a piano, it is the black key between the F and G, called F# (F-sharp). But the note needed to play the G7 chord is a whole step less than G, which is F. So the chord G7 is composed of G, B, D and F. So miracles of miracles, the note needed for the seventh chord to play songs in the key of C is a white key after all!
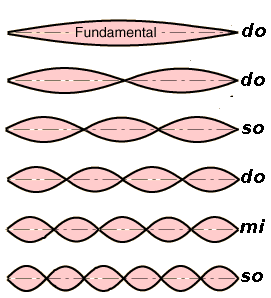
So why does a seventh chord sound so good? Maybe it has to do with what a vibrating string is actually doing. Let's look back at the harmonics of a vibrating string that were shown in Figure 5. When the string is plucked, many higher harmonics are sounded. What is the next one higher? It would have a frequency seven times that of the fundamental. The one after that would be eight times the frequency, so it would again be a do. Thus, the size of the "step" between the seventh and do above would be 8/7. That is very near to a whole step. For example, the whole step from do to re is 9/8, so the two values are very close
As already mentioned, there is no note on the usual scale of seven notes that is a whole step below do, so most music theory stops with the first six wave patterns. But in reality, when a string tuned to C is plucked, one hears one more harmonic, so the ratios are 4, 5, 6, and 7. That is, one hears the entire chord C7 when the a string with a fundamental note of C is struck! That just might be the secret as to why the seventh chord sounds so good in the folk songs.
Here is a note on fine tuning on this diatonic scale. When tuning a string specifically to be used as the extra note in a seventh chord, it technically should be 7/8 of the key note, rather than 8/9 of it. Because I'm unaware of anyone else who has discovered or discussed this problem, the notation used to describe the new note proposed here is a little minus sign as a superscript. For example, the F in the chord G7 is designated by F-. The minus signifies that the note is just slightly lower than usual. We'll see an example of this tuning in the instrument proposed below.
So far it appears to this author that the diatonic scale is superior to the Pythagorean. But in practice, neither is used almost ever today. Both have been replaced by the chromatic scale. So let's look at why.
There are two serious problems with both the Pythagorean and diatonic scales. Both of those scales can be extended to include all of the half step notes with sharps and flats by continuing the processes shown of adding more strings and repeating the intervals. The main purpose for doing that to add unusual notes sometimes needed, such as the note needed for a seventh cord, halfway between la and ti.
The first problem is that the process tends to make a scale with at least 17 notes. Besides the first seven there are five new notes with sharps added to the name, and five more with flats. That gets pretty complicated.
The second problem is that now that we've tuned our instrument with some 17 notes per octave, it can only play in one key. To play in another key, it has to be retuned. Now it is a real pain, so bad as to demand a solution.
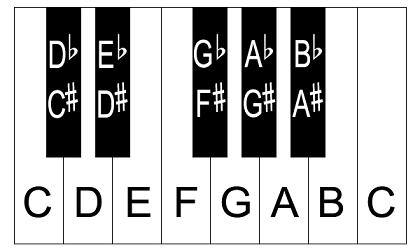 |
The second problem, by far the more serious, is to allow playing in different keys without retuning. Again compromise is the answer. On the diatonic scale, most of the whole steps are not exactly the same size, even though they are very close. The secret to the chromatic scale is that it first defines an octave as before, as exactly a factor of two in frequency, but then it defines the 12 half step notes to be exactly the same size. That one simplification makes everything easy. Every whole step is exactly two half steps, no matter what key is chosen to be do. With that step taken, the song will sound pretty much the same in any key.
|
 |
The international standard for tuning all instruments worldwide to the chromatic scale for nearly a century has been to tune the note A above middle C to 440 Hz. Before that there had been a movement to set the anchor point at middle C being 256 Hz, because that seemed so mathematically nice, it being a power of two (256 = 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2), which is the basis of octaves.
Today there are many musicians wondering whether A being 440 Hz was such a great choice. There is a lot of work being done suggesting that A = 432 leads to a much more pleasing sound. The interested reader can look this work up on the Internet, and even find programs to change your .mp3 music from 440 down to 432 Hz. People take pictures of wave patterns found in nature of things responding more favorably to the 432 value.
So far in this article, nothing would differentiate between these two candidates for best anchor note, but a possible deciding factor will be discussed next.
Thus, there is no argument about the great simplicity and efficiency of the chromatic scale. But the question remains, what is the best tuning of a lyre, where the player is content to play simple songs in only one key, but would like the best possible sound and harmonious tuning. So let us pursue that point.
 |
Before going on, it should be pointed out that if we get excited about the integer numbers being nifty, it must be remembered that they are measured in cycles or vibrations per second. If the second is not a natural unit of time, then there may be no meaning in the numbers. Keeping that in mind, the numbers look great, which to me is a vote for the second being an excellent choice for a unit of time.
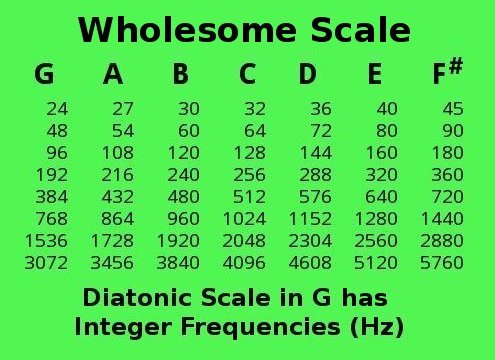
Announcing the discovery of these integer frequencies is really the whole point of this paper. All the rest was context to show how it all fits together. These integer frequencies may or may not sound better, but to me it is mathematically amazing that starting from what some claim is the best value for one note A, all the other values come in whole numbers on a diatonic scale. In the chromatic scale, the only notes which have integer frequencies are the A notes in various octaves.
Because the frequencies are all whole numbers, and because many researchers conclude that a scale based on A being 432 is more wholesome and peaceful to listen to, it is here proposed that the diatonic scale in G major be called the "Wholesome Scale".
So how can we use this new Wholesome Scale, which can only play songs in the key of G? Instruments like a harp or lyre come to mind, with fixed tuning for each string. That is of course why a lyre has been used in the illustrations. A piano could also be tuned this way, but it could only play in one key. But a guitar would not work because it does not have one string per note. The guitar frets used for different notes are for a chromatic scale only.
Traditional harps are tuned with one string for each of the seven notes in a scale. (Foot pedals handle the sharps and flats.) But since the proposed instrument can only play in one key, it would be nice to arrange the strings in the very pattern illustrated in Figure 6 which was used to derive the diatonic scale in the first place. That is, one would already have the three basic chords to play a lot of songs in the key of G. One could just strum three strings in a row to get the desired chord. This would be very easy, not requiring any practice of finger chords as for a guitar.
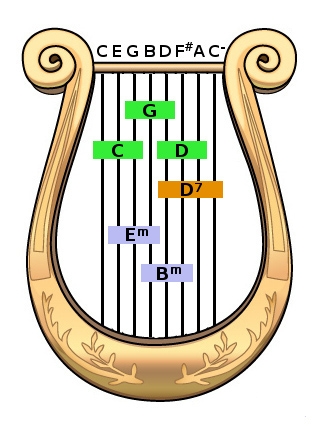 |
Now for a little more practical music theory. If there are three chords, then the progression is usually G to C to D or D7 and back to G. If there are four, then most often they are G to Em to C to D7 and back to G. The little "m" means a minor key. It tends to be a chord that sounds a little sadder than the major cords which is what all the others we've been talking about are called. Each key has what is called a "relative minor" and E is the relative minor of the key of G. This four-chord pattern is the most common for the folk songs of the 60's. One variation, which is in some of the best songs, is to replace Em with Bm, which is the relative minor of the key of D. If all that sounds too tricky, just trust me for now that those are the most common chords for simple songs in the key of G.
| |||||||||||||||||||||||||||
The note for each string is also shown in Figure 10. The first and last C-strings are two octaves apart, just as are the first and last strings on a guitar. Table 5 gives the exact integer frequencies for both a low version (tuned for the same range as a guitar), and for a version one octave higher. That latter does not require such long thick strings, like guitar strings. And for those who are A = 432 aficionados, you'll be happy to know that the A string is at exactly 432 Hz in the low version.
Note that the last entries for the C- in the table are for 504 rather than 512 (the usual C) and 1008 rather than 1024. Those values were calculated by taking 7/8 the frequencies of the note D one step higher, which is appropriate for the seventh chord in the key of D, as discussed above in Section 1.4. They also are whole numbers.
 |
Secondly, it needs eight strings. If it weren't for that, a regular guitar could be a candidate. There are eight-string guitars, but they are mostly electric. But one possibility is the Puerto Rican Guitar (See Figure 11). It has ten strings. They are arranged in five pairs but they should be easy to separate.
If a guitar-like instrument is chosen, one big advantage is that one could easily change keys by using a capo on the frets as described above, because all the strings would change frequency by the same ratio. That is, the frets would only be used to play in a different key signature; they would not be used for fingering different notes. The different keys would lose the integer frequencies, but that might be acceptable.
Thus, at the time of finishing this paper, the Puerto Rican guitar looks like a great candidate, if modified and tuned to be played like a harp. Perhaps it could be called a "guit-harp". The name "harp guitar" already refers to a different instrument.
It is shown that if a diatonic scale in the key of G is constructed based on A above middle-C being tuned to 432 Hz, that all of the frequencies in Hz for the entire range of a piano have integer values. That is such a striking result that it is proposed that the scale be given the name "Wholesome Scale", referring both to the whole numbers and the feeling claimed for a scale based on that frequency. A possible 8-string "guit-harp" is proposed to be tuned to this scale, based on tuning a Puerto Rican guitar and playing it like a harp. Plucking different sets of strings allows playing six of the most common chords to accompany simple songs. The key can be changed with a capo behind the appropriate fret.