by John P. Pratt
9 Mar 2020, 1 Prime (V)
©2020 by John P. Pratt. All rights Reserved.
|
1. The Enoch Calendar 2. Calendar Model 2.1 Intercalation Cycle Use 2.2 Leap Week Placement 2.3 Start Date 3. New Results 4. Conclusion Notes |
A sacred calendar is described in the Book of Enoch, but the method of adding leap weeks was not included. Twenty years of research leading to various mathematical models of the Enoch Calendar, including just when leap weeks should be inserted, have been advanced in my work,[1] but always with the disclaimer that it was a "work in progress" because it was known to be imperfect. For example, two chronologies have kept on my website which include the Enoch Calendar: (1) the Religious Chronology lists the dates which are believed to be correct and (2) the Complete Chronology lists the dates calculated by the computer model. Those two chronologies have never agreed for the Enoch Calendar, because the method of adding leap weeks has not been perfectly known.
Recent advances in the Star Calendar,[2] which also is described in the Book of Enoch and uses exactly the same scheme of inserting leap weeks, have provided more known anchor dates. That new information has been sufficient to resolve the uncertainties and has finally led to what appears to be the correct model of the Enoch Calendar.
This article is a technical description of exactly how the Enoch Calendar works. It is not intended for the casual reader, but for those wishing to understand details of this sacred calendar, enough to enable implementation of a computer model. If, however, a reader is interested only in what new results this model produces, one can skip direction to Section 3 for a summary.
The serious student should read the earlier articles referenced in endnote 1 for background. Only the final results are summarized here.
 |
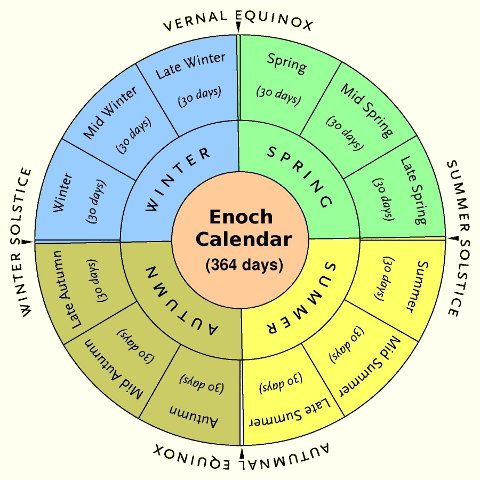
The months are all named for the season, with the first day of the year being on Sunday "1 Spring", and the next day 2 Spring, etc. Each month has 30 days. The last day of each quarter, always being a Saturday, is actually named as the "zeroth" day of the next quarter, and is either an equinox or solstice day. For example, the day preceding 1 Spring is "0 Spring" and is the Spring Equinox day. Thus, all four equinox and solstice days fall on Saturdays. This results from each quarter of 91 days being exactly 13 weeks (7 x 13 = 91, 4 x 91 = 364).
The second two months of each quarter are named "Mid" and "Late" before the season name. Thus, the three months of spring are Spring, Mid Spring, and Late Spring. That same pattern is followed for Summer, Autumn and Winter (See Figure 1).
All holy days fall on fixed days of the year. They are not mentioned in the Book of Enoch but rather are derived from comparison to the Hebrew Calendar holy days. There are two levels of holy days: major and minor. The major holy days are Spring Equinox (0 Spr), New Year (1 Spr), Consecration (10 Spr), Passover (14 Spr), Easter (15 Spr), End Passover (21 Spr), Firstfruits (4 LSp, being 7 weeks after Easter), Summer Solstice (0 Sum), Summer (1 Sum), Autumn Equinox (0 Aut), Trumpets (1 Aut), Atonement (10 Aut), Tabernacles (14 Aut), End Tabernacles (21 Aut), Winter Solstice (0 Win), and Winter (1 Win). Except for the season markers, these days correspond to those of the Hebrew Calendar.
The minor holy days are the first days of the other eight months, along with Lamb Day (13 Spr), Raven Day (14 Sum), Decision Day 1 (17 Sum), Decision Day 2 (24 Sum), Decision Day 3 (1 MSu), Summer Fast (9 MSu), Deluge (17 MAu), Lights (24 LAu), Winter Fast (14 Win), Trees 1 (14 MWi), Trees 2 (15 MWi), Esther 1 (14 LWi), and Esther 2 (15 LWi).
 |
There is, however, also one mysterious rule of intercalation given in the text. It is stated that "the moon brings on all the years exactly" (Enoch 73:13). Just what does that mean? To my knowledge, to all other researchers it has meant that the verse should be ignored, because it is obviously a solar calendar! In my work it has been interpreted to mean that New Year's Day is nearly always on the Sunday after the true equinox, but in the case of the Enoch Spring Eguinox day falling very near the true equinox, if delaying that day for one week would cause the new moon to fall very near the Equinox Day, then the year is delayed a week.
Such a delay by a week should not be too distressing because the Spring Equinox always falls on a Saturday, ideally on or after the true equinox. That precise equinox can fall on either 19, 20 or 21 March, but usually on 20 March. Thus, even without that delay for the moon, the Spring Equinox on the Enoch Calendar can fall anywhere from Sat 19 Mar (if the mean equinox is on Sat 19 Mar) to Sat 27 Mar (if the mean equinox is on Sun 21 Mar). That is 9-day spread, even if the moon is not considered at all! It is similar to how the beginning of the Hebrew year has a spread of over 30 days because it must occur near a new moon.
All of the sacred calendars in my work use the average positions of celestial objects, not their actual locations as determined by observation. For example, if you look at a wall calendar with the true phases of the moon shown, you will see that they are not equally spaced. Some are separated by six days and some by eight. For the purposes of all sacred calendars, including the traditional Hebrew, the apparent positions of the sun and moon are calculated as if they move in perfect circles around the earth, with every phase and month being exactly the same length.
To understand what Enoch means by using the moon to begin all the years with precision, one needs to know just how much emphasis should be given to the sun and how much to the moon. That balance point is what has taken two decades to determine!
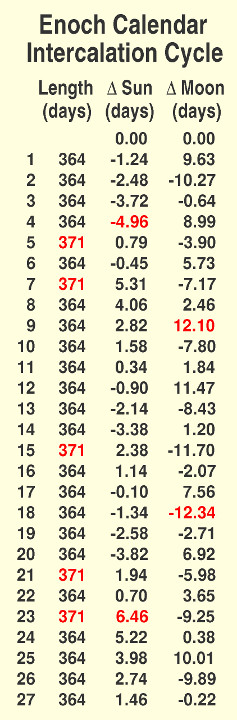
It was discovered early in this work that there is a 27-year cycle after which the day of the solar year is nearly the same and the moon is also very near to new on the first day of the cycle. This cycle can be used to calculate the Enoch Calendar! Table 1 shows the version of this cycle used previously to this article. Note that at the top, the beginning day of the sun and moon are both assumed to be 0.00. That means that the sun is exactly on a Saturday spring equinox and the moon is exactly new. Each line shows the error in days of both sun and moon at the end of that year. For example, at the end of the first 364-day year the solar error is -1.24 days. That is because the true year is 365.24 days long, so after a 364-day year, the New Year's Day will drop by 1.24 days in the true solar year. The third column shows the same error in the 29.53-day lunar cycle.
Now look at the bottom line of the table. After 27 years, the solar day is higher by 1.46 days and the lunar only about a fifth of a day short of being exactly new. That line partially fulfills Enoch's rule that a new moon (that year) should bring in the year exactly! As for the sun, if the cycle began on an Enoch Spring Equinox day on Sat 22 March, then the next 27-year cycle would begin on a Sat 23 or 24 March (1.46 days later). At the end of that second cycle, the sun is still within 2.92 (2 x 1.46) days of the beginning day, and the moon within -0.44 days. Because there is already a 9-day range for the Spring Equinox, this cycle can be repeated a third time. A third cycle would begin on Sat 25 March 2.92 days later on 25 March. In this manner, the table can be used for up to three cycles in a row, ending with a total error of 3 x 1.46 = 4.38 days. That means a fourth cycle would begin on either 26 or 27 March. If the were repeated much longer with no adjustment, the Enoch Spring Equinox would soon occur in April, beyond what is acceptable!
Let us now look at the three requirements to use such a table to calculate any date on the Enoch Calendar.
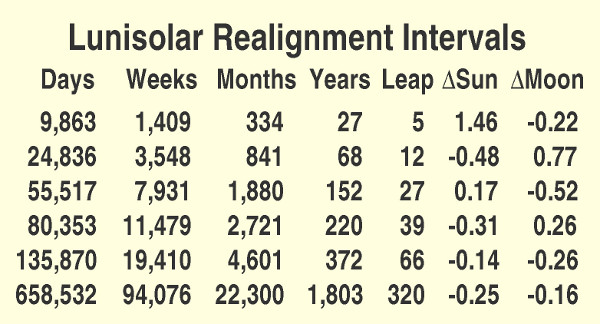 |
The way that most of the sacred calendars which need to intercalate function is that a cycle is used until it gets too far off and then it breaks somewhere and starts over. This is usually done in an elegant manner, meaning the smallest number of breaks or forming a larger pattern. Table 2 shows the units of time called "realignment intervals" when the average periods of the cycles of the sun and moon can both best be measured in weeks.[4]
One of the realignment intervals for the solar year and lunar month is 1,803 years, as shown on the last line of that table. That is a perfect fit for four "Great Seasons" of 1,803 years each, for a total for all of history ("one year with God") being 4 x 1,803 = 7,212 years. That is, the entire history of the family of Adam from the Garden of Eden to the Final Judgment of about 7,200 years can be divided into four equal "Great Seasons" of 1,803 years each![5]
 |
There is a "little season" of about 70 years before 4000 BC while Adam was in the Garden of Eden and there will be another "little season" of about 150-200 years after the Millennium ends about AD 3000. Adding those two little seasons to 7,000 years of history yields about 7,200-7,300 years. That means an ideal period for history should start near 4100 BC and end near AD 3200.
A next step to discovering an elegant pattern of intercalation is to find shorter realignment intervals which can be chained together. The simplest and most common pattern used in sacred calendars is to use two intervals, one slightly long and the other slightly short and alternate them to form larger, more accurate, units. For example, from Table 2 it is seen that alternating periods of 68 years (second line in "Years" column) and 152 years (3rd line) lead to the even more accurate period of 220 years because their errors tend to cancel.[7] Then adding the 152 to the 220 leads to the next period of 372 years.
Those results lead to an elegant pattern indeed, which may well be a witness that it was all designed at the creation. Simply alternating the periods 68 and 84 years leads to: 68 + 84 + 68 + 84 + 68 = 372. That pattern gets more accurate as it progresses because 68 + 84 = 152 and then 152 + 68 = 220. Then a series of those 372-year periods can be repeated to reach the desired 1,803 year result. That is accomplished by cycling through four 372-year periods in a row, and then truncating a fifth such period after 315 years, for a total of 1,803 years (4 x 372 + 315 = 1,803). Then the next 1,803-year Great Season begins, even as there was a similar 1,803-year Great Season preceding it. That is perhaps the most elegant possible intercalation method.
This intercalation method has been known for 17 years but it did not yield the correct results, so it has not been used! Thus, the previous models were known to be wrong and it was still a work in progress. The reason it failed was because the wrong intercalation table was being used (Table 1), as is now discussed.
 |
The simplest way to place the years, to minimize the error in the solar day only, would be to space the leap years evenly throughout the table, about every five years. That would keep the beginning of the year as near to the first Sunday after the equinox as possible.
It was learned long ago in my work that in such cases, the Lord loves variety! Intercalation is rarely done to make every year the same. There will always be a spread of the beginning day of the Enoch year over at least nine days, as discussed above, so why not make that spread a little bigger to also align with moon? That is what the Book of Enoch states to do, which results with the Enoch Calendar aligning more with the Hebrew Calendar where years begin at a new moon.
Given that it is believed that the Lord created the Enoch Calendar and designed the solar system, it is expected that the calendar will be elegant as mentioned above. With that in mind, in order to reduce the vast number of possible tables, let us assume the units of 68 years and 84 years are used as described in Section 1. What are the implications of using those two periods to the requirements for placing the leap years in the intercalation table? Let us consider each those cases separately.
In order to count 68 years continuously with a 27-year table, we must make sure to have the right number of leap years in that interval. The 27-year table requires 5 leap years in it. A series of 68 years must include exactly 12 leap years. Twice around the 27-year cycle gives 54 years with 10 leap years. A 68-year sequence requires that there be exactly 2 more leap (12 total) in the first 14 years of the table, at which time the 68 years will end (27 + 27 + 14 = 68).
Similarly, the sequence of 84 years requires 15 leap years. Three times around the cycle is 81 years which already has 15 leap years in it. That means that there cannot be a leap year in the first three years of table where the last years of 84 will be completed.
Table 1 at the beginning of the article shows that after the first three years with no leap year, the day in the true solar year has dropped by nearly four days (-3.72 days). That means that the first day of the cycle should most likely begin on a Sat 23 Mar or Sat 24 Mar so that the fourth year will have the Solar Equinox day fall on 19 Mar or 20 Mar (not earlier than the equinox). Thus, this simple consideration is a good indicator of the type of day to be sought for a beginning day. Table 1 does not have a leap year in line 4 but in 5. The lowest day of the cycle follows the fourth year being 5 days earlier than the beginning of the cycle. Why was the fourth year not a leap year in Table 1?
Look at maximum solar and lunar errors shown in red in the last two columns of Table 1. That table was devised not only to try to keep the moon near new at the beginning of the year but also to avoid a full moon occurring at that time. If year 4 were a leap year then the next year would have begun with the moon nearly full, so it was skipped. The maximum error in solar days goes from -4.96 days to 6.46 days (in red in second column) for a total spread of 11.42 days! Moreover, remember that in order to have a 84-year period, one must cycle through the table three times (3 x 27 = 81) and go 3 more days into a fourth cycle. As seen by the bottom error of 1.46 days in the second column, each time through the cycle, the beginning is raised by 1.46 days. That means after an 84 years period, the total spread of 11.42 + (3 x 1.46) = 15.80 days! That means that in my former model, on our Gregorian Calendar the Enoch Spring Equinox could occur on anywhere in the 15-day range of 17 Mar to 31 Mar.
Could that range be lessened without losing any elegance? That very wide range occurred because of attempting to avoid full moons at the beginning of the year, which was never required by Enoch. It was my interpretation of what he might have meant. Look at the numbers in red in the third column of Table 1. The phases of the moon range from -12.34 days (which is the same as 29.53 - 12.34 = 17.19) to 12.10 days. A full moon occurs on day 14 or 15, so the good news is that if that table is used, the Enoch year will never begin within two days of a full moon. But the bad news, now recognized, is that the Book of Enoch never made that a requirement! Moreover, that table has never worked! It was that assumed requirement with led to the extra-wide range of the solar error and the wrong intercalation table!
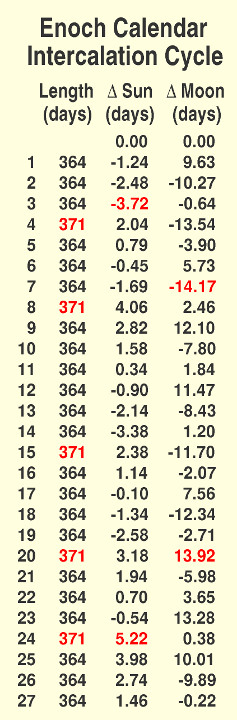
Table 3 is the new proposed 27-year Enoch Calendar Intercalation Cycle. It has two features with better fit the purpose of the Enoch Calendar, which is essentially a solar calendar. First, it has dropped the assumed requirement that the year cannot begin at a full moon. That reduced the spread of solar error to now be only from -3.72 to +5.22 days, for a total range of 8.99 days. That cuts the total solar error down by 2.43 days, so that the total range is only 13.37 days. That is about the minimum possible. If an inelegant method were used of correct the 7-day range with an extra leap week when needed, the range would be at least 14 days (2 weeks).
Secondly, it makes an exception to allow the year to begin exactly on a new moon. Look at the lunar error for year 24. It is only 0.38 days. In other words, the new moon falls exactly on New Year's Day, as mentioned by the Book of Enoch. If it were not for that, the leap year would have occurred in year 25, which would have had the maximum positive solar error of 3.98 days shown on that line. In other words, the reason that the leap year is in year 24 and not 25, which adds 1.24 days to the maximum range, is so that the new moon may fall on New Year's Day at least twice every 27 years (it also occurs on the first day of the cycle as shown on line 27).
Table 3 is elegant, scientifically as accurate as possible, and includes the requirement for the new moon to fall exactly on the new year when possible. Thus, it is here proposed that this intercalation table is now correct.
The last, but also critically important, item required to use the Enoch Calendar Intercalation Cycle is to know on what Saturday to begin! That has been an extremely difficult problem to solve because of the other unknown details of just where the five intercalation years should be placed in the Intercalation Table as well as how to use that table. Those unknowns yielded so many possible combinations that seventeen years elapsed from the first introduction of both the table and realignment periods in 2002 to the first actual proposed implementation in 2019, as noted in endnote 1.
According to the plausible starting date range proposed in Section 2.2, an exhaustive search was made of all possible starting dates from 4200 BC to 3900 BC. The date should be a Saturday, preferably on 23 March, so that the equinox day can drop 5 days after the first three years of the Intercalation Table, and still be on or after 19 March. Each time through the 27-year cycle raises the equinox day so the lowest day ever used for the Spring Equinox should be 18 Mar on the first time through the cycle, that is, on only one day every 68 or 84 years! All others will be on 19 Mar or later.
Moreover, the beginning day should have the moon very near new.
It turns out that the best day scientifically, Sat 23 Mar 4055 BC (Gregorian) with the moon at only 0.2 days past new, is also the correct one to fit all of the known anchor dates! That means that not only is it scientifically sound, but that the Lord is actually using it to schedule sacred events! That is the reason it has been so important to get the correct model for the Enoch Calendar!
This article is now believed to have comprehensively described the Enoch Calendar sufficiently to calculate every day in history. It now appears to be finished, and no longer a work in progress!
The results of discovering this new model are more far reaching than simply finally having a computer model which actually produces the already known right answers for all known anchor dates. It also leads to the discovery of several more important dates of other known sacred historical events now also falling on holy days on the Enoch or Star Calendars. These constitute even stronger witnesses of the accuracy of these dates. Because this article is targeting only those who wish to understand the inner workings of the calendar, only a brief summary of those new dates is given here.
Both my Religious Chronology and the Complete Chronology have been updated to reflect the results of this new model, so they both should agree on every Enoch and Star Calendar date listed. Those new postings show all of the new discoveries, but do not highlight the improvements over the last model. Here is a brief summary of improvements over the last published version of the Religious Chronology (1 Jun 2019). Remember, these are all in addition to calculating all of the anchor dates correctly, which had never been accomplished before!
Most of the following newly discovered sacred days fall on the Star Calendar, so keep in mind that those dates are calculated with exactly the same intercalation pattern as the Enoch Calendar!
Dan and Judah. Dan, son of Jacob, whose name means "Judge", is now born on the Star day named "Judge." His brother Judah is now born on the day named "Lion", matching his blessing which describes him as a young lion (Gen. 49:9).
 |
King David. The day on which David became King of Israel is now on the star day Splendor (Vega), one of the brightest stars in the sky.
Lehi's Departure. The day already proposed for the departure of Lehi from Jerusalem now very appropriately falls on New Year's Day on the Star Calendar. Moreover, the proposed date of Nephi's departure also now falls on the holy star day Elder (Alcyone) in the Pleiades.
Mary's Visit to Elizabeth. This sacred visit not only occurred on a star day associated with John the Baptist on the Uniform Star Calendar, but now it also occurred on a holy star day on the usual Star Calendar.
John the Baptist. The birth of John the Baptist now occurs on the holy star day of the Centaur, representing being both in two different communities, such as Jewish and Christian. In all previous models, there was no star of the Centaur constellation at all in the Star Calendar because it was not known if he were good or evil because he is located down in the evil area of the sky. Now it is understood that the Centaur condescends to go to that region to slay the evil Wolf, being Rome. In the recent update of the Star Calendar, seven stars in the Centaur replaced seven stars in the skirt of Virgo.
Infant Jesus. The day on which baby Jesus was presented to the priest at the temple on His fortieth day was already known to have occurred on Easter Sunday on both the Uniform Hebrew and Uniform Jubilee Calendars, and the day 1 Jeshua (Jesus) on the Priest Calendar. Now it also occurred on the day 22 Taurus, the star day of Fulness (Rigel in Orion), one the brightest stars in the sky. What makes it more interesting is that day was also 22 Aries on the Uniform Star Calendar. The day 22 Aries was Easter on the Star Calendar and 22 Taurus is Easter on the U. Star Calendar, so it is as if those two Easter days were reversed.
Temple Burned. The burning of the temple in AD 70 occurred on the star day Dragon (Thuban in Draco).
Priesthood Restoration. The Aaronic Priesthood was restored on the priesthood star day Elder (Alcyone) which was also the major holy day Firstfruits on the Star Calendar. Moreover, the higher priesthood of apostles was restored by Peter, James, and John on the star day Fulness (Rigel), also a major holy day, so named to refer to fulness of the priesthood. In previous listings, those two dates for the priesthood restoration were chosen because of being major holy days on the Mars Calendar, which is a priesthood calendar. Those days had no priesthood significance on the Star Calendar, but now they do!
Book of Mormon Witnesses. Now the already proposed day of the Three Witnesses viewing the Plates of Mormon occurred on the major holy day the star Scorcher (Sirius, the brightest star in the sky), whereas it was nothing special before. Moreover, the day of the Eight Witnesses now occurs on the star day Warrior (Castor), also a major holy day. In the former model, neither were major holy star days.
The basic elements of the Enoch Calendar intercalation with entire weeks were discovered in my work in 2002. They include a 27-year intercalation cycle, breaking that cycle at key locations in order to end on both solar and lunar realignment intervals with the week, and determining the correct beginning date. Countless attempts have been varying all of those parameters by placing the required leap years in different places in the table, stopping the cycle at different points, and using different starting dates, but none have succeeded in producing an acceptable model.
About 50 anchor dates have been known in religious history because of the witness of several other sacred calendars all having simultaneous holy days on those events. Until now, none of my attempted computer models correctly calculated all of those dates on the Enoch Calendar. Thus, two chronologies have been maintained on my website: one of what I believed were the correct dates and one which were my best calculated dates. It has always been known that the models were incorrect.
In this final attempt, no model was used which was known to be wrong. That meant using only the elegant 68-84-68-84-68 year pattern because it was esthetically pleasing, as well as scientifically most accurate. Using that pattern required modifying the Enoch Calendar Intercalation Cycle to fit all of the known dates. It turned out that the former models had been based on misinterpreting one instruction in the Book of Enoch. When that false assumption was dropped, the model finally worked. The already accepted starting date was verified to be correct. With the new intercalation table, all fifty of the anchor dates were correctly calculated as well as many new anchor dates on the Star Calendar, which uses the same intercalation scheme. Moreover, several new holy days on other sacred events were also discovered as additional witnesses that the Enoch Calendar intercalation algorithm is now known with certainty.
The Enoch Calendar is a witness of the hand of the all-knowing Creator in the formation of the solar system because He appears to be using that calendar for timing certain sacred events. Without it having been intelligently designed, the intercalation could not have been made so elegantly. Truly the sun, moon, and stars all testify of the existence of an all-wise Creator!